Grasshoppers, Quantum Physics, and the NSF: Professor Takes on ‘the Grasshopper Problem’
Imagine that a grasshopper is sitting on a lawn. It jumps. What is the probability that it lands back on the lawn?

The question is known as “the grasshopper problem,” and the answer is more complex than you might think. So difficult, that Olga Goulko, an assistant professor of physics at UMass Boston, was just awarded $300,000 from the National Science Foundation (NSF) to figure it out.
Goulko’s approach to answering the question starts relatively simply: by attempting to guess two unknown variables. The first is in what direction a random grasshopper will jump on a random hop. The second is what the shape of the lawn that they are hopping on is.
But Goulko, an expert in quantum many-body physics, condensed matter theory, and computational methods, is not asking grasshoppers about their next jumps or readying her tape measure for neighboring lawns. Instead, in trying to solve the problem, she and her team of researchers are using statistical physics and quantum information, two of her expertise that uniquely apply to the variables that must be solved to answer the question.
“This is very much a marriage of quantum information and pure mathematics, because the question itself is a mathematical and statistical mechanics question, and then we use computational approaches [and quantum information] to study it,” she said, explaining her team’s models to solve the problem. “In fact, the computational approaches are pretty simple.”

One of the computer renderings from Goulko's team, modeling where a grasshopper might jump. Courtesy of Olga Goulko.
But why study the grasshopper problem? It’s a natural question for Goulko’s work, but one that has a good answer.
“What the work does is it studies a somewhat unusual, more general class of Bell inequalities that involve a higher degree of randomness than the standard Bell inequalities that people may learn about in physics class,” she said, noting the practical application of a theoretical problem.
Another advantage to the grasshopper problem is that it is uniquely suited for people new to the physics world. By taking complex theories and statistical models and boiling them down to solve something tangible, Goulko and her team are creating opportunities for young people in the field to learn about computational statistics and quantum information.
“It's a really great way for … beginning students to get into computational physics and to get to know programming and algorithms,” said Goulko. “It's very quick to code, even undergraduate students [are able to do it].”
The grant from the NSF will be distributed over three years and pay for undergraduate and graduate assistantships that will work alongside Goulko on solutions to the problem. It will also fund travel for Goulko and colleagues from other universities who are studying the problem, including Adrian Kent, from the University of Cambridge, and Dmitry Chistikov and Mike Paterson, both from the University of Warwick.
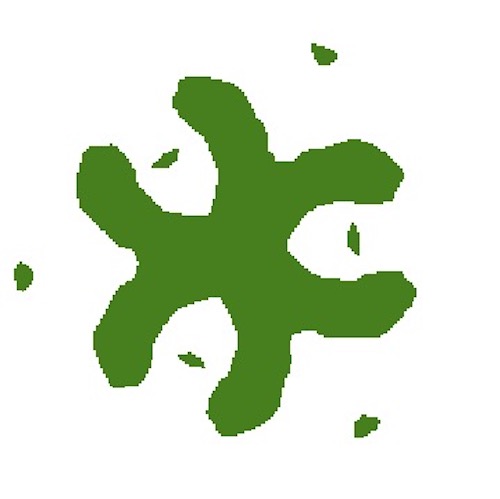
One of the shapes that Goulko's team have found have a high probability of the grasshopper landing on them. Courtesy of Olga Goulko.
So, what shape does the lawn have to be to solve the problem?
“It's a very open-ended question, and there's so many different shapes, but we have many numerical results already,” said Goulko. “What is so interesting and surprising about this problem is that the numerical results don’t at all look like what we originally expected. There is a great variety of shapes with unusual symmetries.”
